center of mass concepts

center of mass Concepts

Today our topic is about center of mass very important topic for physics, If you will understand this topic well then you can command over the mechanics complex and difficult problems, With the help of Center of mass concept, You can solve the numerical problems easily. This center of mass concept is used broadly in rotational and transnational mechanics, Center of mass has great importance to understand concept of mechanics, Hence lets start center of mass basic meaning and its concepts.

center of mass definition physics  

Center of mass meaning mass of center not the geometric center only mass center, Hence center of mass is that point, Where we suppose whole of the mass is concentrated, Which can be lies inside the body or lies outside the body. some important facts about center of mass.

Some important facts about Center of mass 

1 Center of mass is an imaginary point ( Why imaginary point ? because it is not necessary there may be something it may free space, Hence center of mass point can be free space no anything at that point so it is called imaginary point )

2 Center of mass may or may not be located on system (why it lies outside system ? because every body or system want stability and minimum energy, hence Center of mass lies outside to balance system )

3 Whole mass of the body system can be assumed to be concentrated on center of mass ( why that ? because when body pass with any process considering as whole mass the output result will be same as when considering pass through center of mass).

                                                                 

Center of mass Concepts

                                                          

Why Center of mass need to study ?    

All rules are valid for point mass only, There are so many rules in Physics which is only valid for point mass , As for example Newton's second law F = ma, This rule is valid only where m is point mass,And a is acceleration of center of mass, We also use equation p=mv where v is velocity of center of mass Second example Newton's law of gravitation where force of attraction between two masses F = Gm1m2/r²  This law is also valid only for point masses.

But in general practical life example any body has some geometrical shape and big size except particle electron,proton and neutron all body having some shape and size which can't be like point mass because it occupies big space, So we need a point of mass such a way that where whole body of mass is concentrated, Replacing big body as point of center of mass such that the behavior of body should not change . Hence we need to study Center of mass.So in Physics rules a big body we have to assumed a point of mass, Which is consider as center of mass, then we have to find the location of center of mass, where  whole body of mass is concentrated.Hence concept of center of mass came in picture, So we need to find the location of center of mass in this topic as above picture shown.

from above picture of table a piece of paper is kept if we want to lift the paper vertically, tie four string on the corner of square size paper, and hence we can lift the paper vertically, But other second method find the center of mass of the square paper and apply directly force vertically at center of mass, paper will be lifted vertically without changing it behavior using only a single point called center of mass. This is the application of center of mass.

How to calculate center of mass ?    

Now here we will see how to calculate or find out center of mass for any body or point mass it is written in short COM(Center of mass).

Suppose there are many masses like m1,m2, m3........  then we need a Cartesian plan to find out the center of mass of the system see below picture.

                                                               

  

   Now technique for finding center of mass try to place the body on  Cartesian plan, So that we get maximum number of zeros or maximum symmetry so that it will be easy to find out center of mass of the body or system.

How can guess center of mass position of a body ?    

As we have discussed position of center of mass of a body depend upon shape of the body.

The position of center of mass will shift towards heavy mass of the body,So you will get center of mass towards heavy mass of the body.

Center of mass divide length in opposite ratio of mass this is important point about center of mass.Center of mass shift closer to heavy mass.

Center of mass is independent of coordinate system, In other words center of mass of the body does not change with respect to body,Whenever coordinate system is change.

Try to find symmetry to find center of mass, See below picture for above mentioned point.

centre of mass problems

                                                                 

  

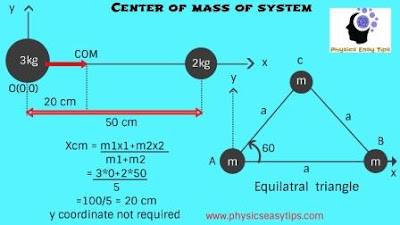
 Here for Equilateral triangle we have to find center of mass first find out coordinate of all three mass point A, B, C .

As from figure coordinate of A(0,0) , B(a,0) for coordinate of C apply geometry x coordinate will be a/2 now for y coordinate sin60⁰ = y/a so y = asin60⁰ =   √3*a/2 hence coordinate of point C(a/2, √3*a/2) .

Now use the above discussed formula 

Xcm = (m1x1+m2x2+m3x3)/(m1+m2+m3) = (m*0+m*a+m*a/2)/3m =  a/2

Ycm = (m1y1+m2y2+m3y3)/(m1+m2+m3) = (m*0+m*0+m*√3*a/2)/3m = a/2√3 .

Hence Center of mass for equilateral triangle is (a/2, a/2√3).

Try to use symmetry to find center of mass

With the help of symmetry complex problem can be solved by easily so whenever any system of mass is given and asked to find the center of mass then try to use symmetry as possible lets see below hexagonal problem.

                                                               

From the above hexagon question applying symmetry see how it is easily solved, so you have to use the symmetry to find center of mass.

Now after applying symmetry all masses located on a single axis x, Where coordinate of all masses are now known, Hence to find center of mass we can apply formula.

Xcm  = (m1x1+m2x2+m3x3+m4x4)/(m1+m2+m3+m4)

Xcm  = (m*0+4m*a/2+2m*3a/2+2m*2a)/(m+4m+2m+2m)

Xcm  = (0+2ma+3ma+4ma)/9m = 9ma/9m = a

Hence center of mass of this hexagon will be COM(a,0) here y coordinate is zero .

Here center of mass located on x axis at a distance a from origin.  

We will continue this topic in next post, I hope that you have enjoyed learning Center of mass, If you like please comments and share on social media,Thanks for reading and sharing keep learning more and more.

Dated 19th Oct 2018