Hello Friends,

Dimensional analysis of physical quantities

Today our topic is dimensional analysis, we have study previously seven fundamental quantities and two supplementary quantities and also there are so many derived quantities with the help of fundamental quantities, now what is dimension ? answer dimension is characteristics of physical quantities, dimension is attached with physical quantities whether it is fundamental physical quantities or derived physical quantities for both type of quantities it is applicable in other way we can say that dimension describe how many parameter are involved in a physical quantities like area, volume,force, energy and many more all are physical quantity hence all will have dimension, any physical quantities expressed in fundamental quantities and this fundamental quantities is know as dimension of that physical quantities and how many times fundamental quantities are used is the number of dimension of that physical quantity.Fundamental quantity and its multiple are dimension of a given physical quantity.

                                                                             

Dimensional analysis of physical quantities

                                               

 Example Area has two dimension of length because we measure area length*length hence it has two dimension of length, similarly volume has 3 dimension of length because volume is define as length*length*length above picture shows. here length is fundamental quantity multiply 2 times for area and 3 times for volume, it is not necessary only one fundamental quantity used for dimension more than one fundamental quantity is used to made dimension of a physical quantity like density it is define as mass per unit volume that is d = mass/volume here d is density here two fundamental quantity is used mass and length for dimension of physical quantity density one dimension of mass and 3 dimensions of length.

Dimensional Formula writing dimension quantity with its sign and multiplicity see below some example. hence for any physical quantity four information is important that is quantity, formula, dimension, unit. so whatever quantity we will study these four information must know.

Quantity	Formula	Dimension	Unit
Area	Length*Length	L²	m²
Volume	Length*Length*Length	L³	m³
Density	Mass/Volume	ML⁻³	Kg/m³
Velocity	Displacement/Time	LT⁻¹	m/s
Acceleration	∆Velocity/Time	LT⁻²	m/s²
Force	Mass*acceleration	MLT⁻²	kgm/s² (Newton)

Now for any physical quantity we can made dimension but for that we must know that physical quantity physical formula otherwise we can't made dimension now how physical formula is made answer is definition of physical quantity like speed or velocity is define as distance travel per unit time means physical formula distance/time is speed or displacement/time is velocity. hence with the help of physics definition we made physical formula and with the help of physical formula we made dimension and with the help of both these we made unit so whatever derive physical quantity we will study in each topic we will see its dimensional formula at that time just here seen some fundamental quantity dimension because it is just made by formula and right now we have not studies those formula latter all formula will be cover and it will be easy but remember by heart some fundamental dimension what above we have discussed, some of quantity we will study in mechanics work, energy, power, momentum. now important point is what are advantage of making these dimensional formula answer is we must know the physical quantity we study which parameter effect that physical quantity example force we have seen dimension MLT⁻²  

 it clear that force physical quantity depends upon three fundamental quantity mass, length and time which itself state that if you want to apply force on any heavy body to displace it on short time you need to apply more force because mass is directly proportional distance you want to displace is directly proportional time is inversely proportional that is in less time more displacement need more force so how we made these conclusion about force simple with the help of dimension so it is powerful tool to explain about physical quantity depending parameter, now for velocity we want to move a car distance of 10 m in just 5 sec then its speed will be 10/5 = 2 m/s but we say a heavy truck want to move 10 m in 2 s then what will be its velocity then our answer is same 2 m/s why ? because dimension of velocity is LT⁻¹ which indicate that velocity is independent of mass velocity only depend upon length and time.hence we get a information from dimension which basic quantity effect our given physical quantity.

Use of Dimensional Formula: 

1 Involvement of basic/Fundamental quantities 

2 which quantity effect directly (positive power) and which quantity effect inversely (negative power) 

3 which quantity is more effective by seeing the power take an example of force we know that force dimension is F = MLT⁻² if we increase length 3 times force will increase by 3 times but if we increase time value 3 times then force value will decrease by 9 times because time is negative square here this is important point. Applications of dimension:

1 To Convert unit dimension of a physical quantity never changed its unit may change example we know that Area is length*length its dimension is L² its unit may be km², m², feet², cm², mm² but its dimension will be always L² we know that any physical quantity is written as q = n*u where n is magnitude or number and u is its unit  while changing unit its magnitude n will change but dimension will not change example converting velocity 1 km/ hr to m/s here we will take help of dimension to convert we will write as for conversion n1LT⁻¹ = n2LT⁻¹ here we have to determine n2 hence n2 = 1*(km/m)¹*(hr/s)⁻¹ = (1000m/m)¹*(3600s/s)⁻¹ = 1000/3600 = 5/18 now here unit of n2 is m/s hence (5/18)m/s so we can covert one unit to other unit if reverse m/s to km/hr it will be 18/5 km/hr hence dimension formula is useful for converting unit.hence convert yourself 1 N force to cgs system dynes hints Force dimension MLT⁻² dimension formula will not change because both are force. 

2 To check whether a formula of a given quantity is correct ? when we do experiment of simple pendulum in a lab and calculate its time period and we found its time period T = 2𝛑√l/g 

now to check whether this formula is correct for this we work on a principle called principle of homogeneity this describe that in physics dimension of all terms in a physical relation are same and it is simple rule because 5 kg rice is not equal to 10 m both side unit must be same any equation may be like v = u+at  so all terms dimension must be same  hence now check dimension for simple pendulum time period for this take LHS side dimension that is T¹ now take RHS here 2 & 𝛑   are  number dimensionless 

RHS = ⎣ L/LT⁻²⎦¹÷² = T hence LHS dimension  = RHS dimension so formula is dimensionally  correct.  

Now you can check yourself for equation 1/2mv² = mgh

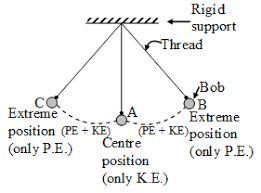
3 To derive a formula how to make a relation between different quantity take an example of simple pendulum oscillation .

                                               

Dimensional analysis of physical quantities

we oscillate this pendulum and note its time period there are so many physical quantity involved in it then we change its length and measure time, next changed mass and measure time again its location is change taken this pendulum on mountain where value of gravity will be change and measure it time and found many relations so what are the quantities we are studying here that is time, length, mass and g acceleration due to gravity these are the quantities related to each other now we want to see what are effect of length, mass and g on time this will be our formula now we express time in terms of length, mass and g written as T𝝰lªmˢgⁿ time is directly proportional to product of length,mass and gravity it can be also written as T = klªmˢgⁿ where k is constant a,s,n are the power which we have to find out value of a,s,n the our formula will be ready now write dimension of equation on both side hence M⁰L⁰T¹  = LªMˢ*(LT⁻²)ⁿ = Lª⁺ⁿMˢT⁻²ⁿ   here k is constant dimensionless now compare power on both side then we will get the equation  s = 0, a+n = 0, -2n = 1, n = -1/2, a = 1/2,  hence after putting value T = k√l/g now here we can't found value of k it is calculated in lab so you can made more formula with the help of dimension but here is some limitation of dimension analysis. 1 we can't find constant value . 2 limitation to three unknown variable because here using MLT only. 3 Limitation to single term equation only example s = ut+1/2at² can't made by dimension this formula because here are two term first ut and second 1/2at² now your dimension analysis is over thanks for reading. Dimensional analysis of physical quantities.